 Systems with Zero as an Eigenvalue
Systems with Zero as an Eigenvalue Systems with Zero as an Eigenvalue
Systems with Zero as an Eigenvalue

We discussed the case of system with two distinct real eigenvalues,
repeated (nonzero) eigenvalue, and complex eigenvalues. But we did not
discuss the case when one of the eigenvalues is zero. In fact, it is
easy to see that this happen if and only if we have more than one
equilibrium point (which is (0,0)). In this case, we will have a
line of equilibrium points (the direction vector for this line is the
eigenvector associated to the eigenvalue zero).
Example. Find the general solution to
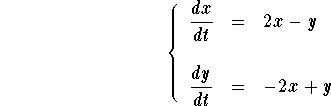
Answer. The characteristic polynomial of this system is
![]()
which reduces to ![]() . The eigenvalues are
. The eigenvalues are
![]() and
and ![]() . Let us find the associated
eigenvectors.
. Let us find the associated
eigenvectors.
![]()
The equation ![]() translates into
translates into
![]()
The two equations are the same. So we have y = 2x. Hence an eigenvector is
![]()
![]()
The equation ![]() translates into
translates into
![]()
The two equations are the same (as -x-y=0). So we have y = -x. Hence an eigenvector is
![]()
Therefore the general solution is
![]()
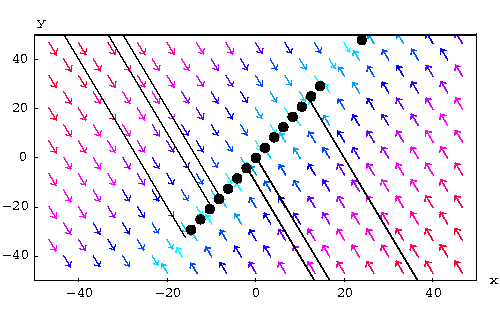
Note that all the solutions are line parallel to the vector
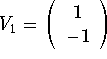 .
When
.
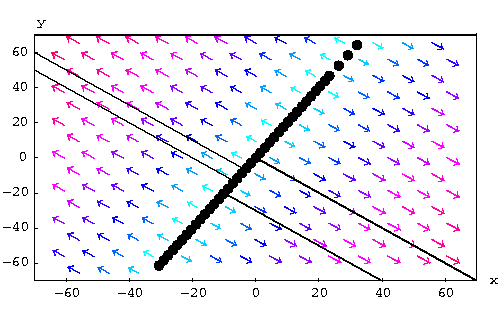
When ![]() , the trajectory goes to infinity. But
when
, the trajectory goes to infinity. But
when ![]() , the trajectory converge to the
equilibrium point on the line of equilibrium points (that is passing
by (0,0) and having
, the trajectory converge to the
equilibrium point on the line of equilibrium points (that is passing
by (0,0) and having ![]() as a direction vector). The picture below
explains more what is happening.
as a direction vector). The picture below
explains more what is happening.
|
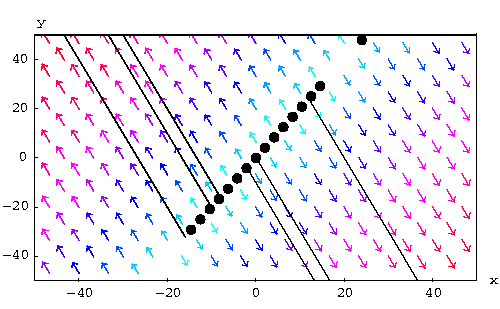
The general case is very similar to this example. Indeed, assume that
a system has 0 and ![]() as eigenvalues. Hence if
as eigenvalues. Hence if ![]() is
an eigenvector associated to 0 and
is
an eigenvector associated to 0 and ![]() an eigenvector associated to
an eigenvector associated to
![]() , then the general solution is
, then the general solution is
![]()
We have two cases, whether ![]() or
or ![]() .
.
|
|

 S.O.S MATHematics home page
S.O.S MATHematics home page Do you need more help? Please post your question on our S.O.S. Mathematics CyberBoard.

Author: Mohamed Amine Khamsi